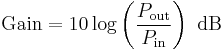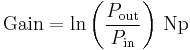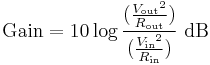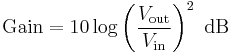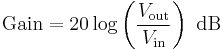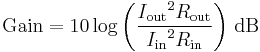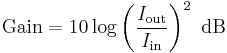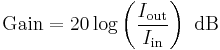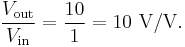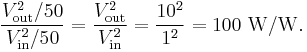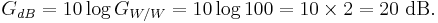Gain
In electronics, gain is a measure of the ability of a circuit (often an amplifier) to increase the power or amplitude of a signal from the input to the output. It is usually defined as the mean ratio of the signal output of a system to the signal input of the same system. It may also be defined on a logarithmic scale, in terms of the decimal logarithm of the same ratio ("dB gain"). A gain greater than one (zero dB), that is, amplification, is the defining property of an active component or circuit, while a passive circuit will have a gain of less than one.
Thus, the term gain on its own is ambiguous. For example, "a gain of five" may imply that either the voltage, current or the power is increased by a factor of five, although most often this will mean a voltage gain of five for audio and general purpose amplifiers, especially operational amplifiers, but a power gain for radio frequency amplifiers, and for directional aerials will refer to a signal power change compared with a simple dipole. Furthermore, the term gain is also applied in systems such as sensors where the input and output have different units; in such cases the gain units must be specified, as in "5 microvolts per photon" for the responsivity of a photosensor. The "gain" of a bipolar transistor normally refers to forward current transfer ratio, either hFE ("Beta", the static ratio of Ic divided by Ib at some operating point), or sometimes hfe (the small-signal current gain, the slope of the graph of Ic against Ib at a point).
In laser physics, gain may refer to the increment of power along the beam propagation in a gain medium, and its dimension is m−1 (inverse meter) or 1/meter.
Contents |
Logarithmic units and decibels
Power gain
Power gain, in decibels (dB), is defined by the 10 log rule as follows:
where Pin and Pout are the input and output powers respectively.
A similar calculation can be done using a natural logarithm instead of a decimal logarithm, and without the factor of 10, resulting in nepers instead of decibels:
Voltage gain
When power gain is calculated using voltage instead of power, making the substitution (P=V 2/R), the formula is:
In many cases, the input and output impedances are equal, so the above equation can be simplified to:
and then the 20 log rule:
This simplified formula is used to calculate a voltage gain in decibels, and is equivalent to a power gain only if the impedances at input and output are equal.
Current gain
In the same way, when power gain is calculated using current instead of power, making the substitution (P = I 2R), the formula is:
In many cases, the input and output impedances are equal, so the above equation can be simplified to:
and then:
This simplified formula is used to calculate a current gain in decibels, and is equivalent to the power gain only if the impedances at input and output are equal.
The "current gain" of a bipolar transistor, hFE or hfe, is normally given as a dimensionless number, the ratio of Ic to Ib (or slope of the Ic-versus-Ib graph, for hfe).
In the cases above, gain will be a dimensionless quantity, as it is the ratio of like units (Decibels are not used as units, but rather as a method of indicating a logarithmic relationship). In the bipolar transistor example it is the ratio of the output current to the input current, both measured in Amperes. In the case of other devices, the gain will have a value in SI units. Such is the case with the operational transconductance amplifier, which has an open-loop gain (transconductance) in Siemens (mhos), because the gain is a ratio of the output current to the input voltage.
Example
Q. An amplifier has an input impedance of 50 ohms and drives a load of 50 ohms. When its input (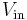 ) is 1 volt, its output (
) is 1 volt, its output ( ) is 10 volts. What is its voltage and power gain?
) is 10 volts. What is its voltage and power gain?
A. Voltage gain is simply:
The units V/V are optional, but make it clear that this figure is a voltage gain and not a power gain. Using the expression for power, P = V2/R, the power gain is:
Again, the units W/W are optional. Power gain is more usually expressed in decibels, thus:
A gain of factor 1 (equivalent to 0 dB) where both input and output are at the same voltage level and impedance is also known as unity gain.
See also
- Active laser medium
- Antenna gain
- Aperture-to-medium coupling loss
- Automatic gain control
- Attenuation
- Complex gain
- DC offset
- Effective radiated power
- Gain before feedback
- Insertion gain
- Loop gain
- Open-loop gain
- Net gain
- Power gain
- Process gain
- Transmitter power output
This article incorporates public domain material from the General Services Administration document "Federal Standard 1037C".